Sersic profile
The Sérsic profile (or Sérsic model or Sérsic's law) is a mathematical function that describes how the intensity 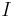 of a galaxy varies with distance
of a galaxy varies with distance  from its center. It is a generalization of de Vaucouleurs' law. J. L. Sérsic first published his law in 1963.[1]
from its center. It is a generalization of de Vaucouleurs' law. J. L. Sérsic first published his law in 1963.[1]
Contents |
Definition
The Sérsic profile has the form
where  is the intensity at
is the intensity at  . The parameter
. The parameter  , called the "Sérsic index," controls the degree of curvature of the profile (see figure). The smaller the value of
, called the "Sérsic index," controls the degree of curvature of the profile (see figure). The smaller the value of  , the less centrally concentrated the profile is and the shallower (steeper) the logarithmic slope at small (large) radii is:
, the less centrally concentrated the profile is and the shallower (steeper) the logarithmic slope at small (large) radii is:
Applications
Most galaxies are fit by Sérsic profiles with indices in the range 1/2 < n < 10. The best-fit value of n correlates with galaxy size and luminosity, such that bigger and brighter galaxies tend to be fit with larger n. [2] [3] Setting n = 4 gives the de Vaucouleurs profile:
which is a good description of giant elliptical galaxies. Setting n = 1 gives the exponential profile:
which is a good description of spiral galaxy disks and dwarf elliptical galaxies. The correlation of Sérsic index (i.e. galaxy concentration) with galaxy morphology is sometimes used in automated schemes to determine the Hubble type of distant galaxies.[4] Sérsic indices have also been shown to correlate with the mass of the supermassive black hole at the centers of the galaxies. [5]
Sérsic profiles provide the best current description of dark matter halos, and the Sérsic index correlates with halo mass.[6] [7]
Generalizations of the Sérsic profile
The brightest elliptical galaxies often have low-density cores that are not well described by Sérsic's law. The "core-Sérsic" family of models was introduced by A. Graham et al. [8] and Trujillo et al. [9] and further developed by B. Terzić and A. Graham in 2005 to describe such galaxies.[10] Core-Sérsic models have an additional set of parameters that describe the core radius and core density.
Dwarf elliptical galaxies sometimes have pointlike nuclei that are also not well described by Sérsic's law. These galaxies are often fit by a Sérsic model with an added central component representing the nucleus. [11] [12]
The Einasto profile is mathematically identical to the Sérsic profile, except that 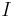 is replaced by
is replaced by  , the space density, and
, the space density, and  is replaced by
is replaced by  , the true (not projected) distance from the center.
, the true (not projected) distance from the center.
See also
References
- ^ J. L. Sérsic (1963), Influence of the atmospheric and instrumental dispersion on the brightness distribution in a galaxy
- ^ N. Caon et al. (1993), On the Shape of the Light Profiles of Early Type Galaxies
- ^ C. Young & M. Currie (1994), A New Extragalactic Distance Indicator Based on the Surface Brightness Profiles of Dwarf Elliptical Galaxies
- ^ A. van der Wel (2008), The morphology-density relation: a constant of nature
- ^ A. Graham & S. Driver (2007), A Log-Quadratic Relation for Predicting Supermassive Black Hole Masses from the Host Bulge Sérsic Index
- ^ D. Merritt et al. (2005), A Universal Density Profile for Dark and Luminous Matter?
- ^ D. Merritt et al. (2006), Empirical Models for Dark Matter Halos. III. Nonparametric Construction of Density Profiles and Comparison with Parametric Models
- ^ A. Graham et al. (2003), A New Empirical Model for the Structural Analysis of Early-Type Galaxies, and A Critical Review of the Nuker Model
- ^ I. Trujillo et al. (2004), Evidence for a New Elliptical-Galaxy Paradigm: Sérsic and Core Galaxies
- ^ B. Terzić & A. W. Graham (2005), Density-potential pairs for spherical stellar systems with Sérsic light profiles and (optional) power-law cores
- ^ A. Graham & R. Guzmán (2003), HST Photometry of Dwarf Elliptical Galaxies in Coma
- ^ P. Cote et al. (2006), The ACS Virgo Cluster Survey. VIII. The Nuclei of Early-Type Galaxies
External links
- Stellar systems following the R exp 1/m luminosity law A comprehensive paper that derives many properties of Sérsic models.
- A Concise Reference to (Projected) Sérsic R1/n Quantities, Including Concentration, Profile Slopes, Petrosian Indices, and Kron Magnitudes.



